C.A. Weidner, S.G. Schirmer, F.C. Langbein, E.A. Jonckheere. Applying Classical Control Techniques to Quantum Systems: Entanglement versus Stability Margin and other Limitations. IEEE Conf Decision and Control, pp. 5813-5818, 2022. [DOI:10.1109/CDC51059.2022.9992337] [arXiv:2207.12385] [PDF:paper]
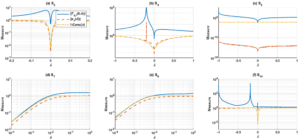Development of robust quantum control has been challenging and there are numerous obstacles to applying classical robust control to quantum system including bilinearity, marginal stability, state preparation errors, nonlinear figures of merit. The requirement of marginal stability, while not satisfied for closed quantum systems, can be satisfied for open quantum systems where Lindbladian behavior leads to non-unitary evolution, and allows for nonzero classical stability margins, but it remains difficult to extract physical insight when classical robust control tools are applied to these systems. We consider a straightforward example of the entanglement between two qubits dissipatively coupled to a lossy cavity and analyze it using the classical stability margin and structured perturbations. We attempt, where possible, to extract physical insight from these analyses. Our aim is to highlight where classical robust control can assist in the analysis of quantum systems and identify areas where more work needs to be done to develop specific methods for quantum robust control.
A video presenting this paper is on YouTube.
This work is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License.