S. P. O’Neil, C. A. Weidner, E. A. Jonckheere, F. C. Langbein, S. G. Schirmer. Robustness of Dynamic Quantum Control: Differential Sensitivity Bounds. AVS Quantum Sci. 6, 032001, 2024.
[DOI:10.1116/5.0196110]
[arXiv:2401.00301]
[PDF]
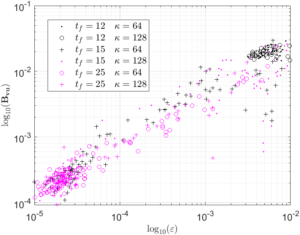
Dynamic control via optimized, piecewise-constant pulses is a common paradigm for open-loop control to implement quantum gates. While numerous methods exist for the synthesis of such controls, there are many open questions regarding the robustness of the resulting control schemes in the presence of model uncertainty; unlike in classical control, there are generally no analytical guarantees on the control performance with respect to inexact modeling of the system. In this paper, a new robustness measure based on the differential sensitivity of the gate fidelity error to parametric (structured) uncertainties is introduced, and bounds on the differential sensitivity to parametric uncertainties are used to establish performance guarantees for optimal controllers for a variety of quantum gate types, system sizes, and control implementations. Specifically, it is shown how a maximum allowable perturbation over a set of Hamiltonian uncertainties that guarantees a given fidelity error can be reliably computed. This measure of robustness is inversely proportional to the upper bound on the differential sensitivity of the fidelity error evaluated under nominal operating conditions. Finally, the results show that the nominal fidelity error and differential sensitivity upper bound are positively correlated across a wide range of problems and control implementations, suggesting that in the high-fidelity control regime, rather than there being a trade-off between fidelity and robustness, higher nominal gate fidelities are positively correlated with increased robustness of the controls in the presence of parametric uncertainties.
This work is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License.