M. Li, F. C. Langbein, R. R. Martin. Detecting Approximate Incomplete Symmetries in Discrete Point Sets. In: Proc. ACM Symp. Solid and Physical Modeling, pp. 335-340, ACM Siggraph 2007. [DOI:10.1145/1236246.1236294] [PDF]
li2007
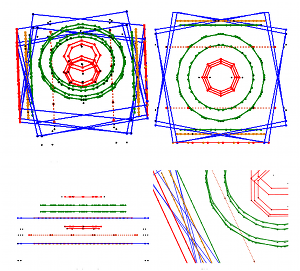
Motivated by the need to detect design intent in approximate boundary representation models, we give an algorithm to detect incomplete symmetries of discrete points, giving the models’ potential local symmetries at various automatically detected tolerances. Here, incomplete symmetry is defined as a set of incomplete cycles which are constructed by, e.g., a set of consecutive vertices of an approximately regular polygon, induced by a single isometry. All seven 3D elementary isometries are considered for symmetry detection. Incomplete cycles are first found using a tolerance-controlled point expansion approach. Subsequently, these cycles are clustered for incomplete symmetry detection. The resulting clusters have well-defined, unambiguous approximate symmetries suitable for design intent detection, as demonstrated experimentally.
This work is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License.